The Quadratic formula is a way to solve quadratic equations, or equations with the power of two. Read on for more information.
The Quadratic Formula[]
The formula is best given in this picture from scientificpages.net. Here it is:
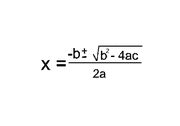
The quadratic formula at its finest.
Derivation of the Quadratic Formula[]
The quadratic formula isn't some magical thing that some ingenius computer made up. The quadratic formula is actually simply the standard form of a quadratic equation (ax^2+bx+c=0) with the square completed on it. Try it out yourself!
Using the Quadratic Formula[]
To use the quadratic formula, you must first put the equation into standard form. For example, 5=6x^2-25x becomes -6x^2+25x+5. Then, you look at the coefficients and constants, and assign the variables to them. For example:
ax^2+bx+c=0
(-6)x^2+(25)x+(5)=0
a=-6, b=25, c=5
(NOTE: The "a" is the number that multiplies the x^2 term, the "b" is the number that multiplies the x term, and finally, c is the constant.)
Using these numbers, you can now plug them into the quadratic formula and simplify.
What to do when the square root comes out negative[]
If b^2 - 4ac, the part of the quadratic formula under the square root comes out negative, then there is no real solution to the problem, because you can't have negative square roots (unless you express it with complex numbers).
Practice Problems[]
This is just a little practice so that you can make sure you understand all of the concepts.
Here are the problems:
- Solve 4x^2+8x+4=0 using the quadratic formula.
- Solve 3x^2-17x-10=0 using the quadratic formula.
- Solve 4x=7x^2-1 using the quadratic formula.
- Solve 100=2x-64x^2 using the quadratic formula.
Answers are at the Q Answer Page.